The Dispersion Relation of the LETG+HRC-S

Sun Mi Chung, Jeremy J. Drake, Vinay L. Kashyap, and the LETG team

August 2004

(This page describes the dispersion relation status as of Aug 2004. A wavelength correction tool, corrlam, based on extensions of the work described here was released early in 2005.)

Summary

The dispersion relation of the LETG+HRC-S is currently known to approximately a few parts in 10000, across the entire LETG+HRC-S wavelength range. With a dispersion of 1.148 Å/mm, the LETG+HRC-S covers a wavelength range of -165 to +175 Å. We have recently corrected the wavelength discontinuities observed between the central and outer HRC-S microchannel plates by effectively enlarging the plate gaps in the data processing software. This change has been implemented in the latest release of CIAO version 3.1. The dispersion relation for data processed with CIAO 3.1 shows an RMS of only 0.014 Å, across the entire LETG+HRC-S wavelength range.

Although the wavelength discontinuities between the central and outer HRC-S plates have been corrected, there still remain non-linearities in the dispersion relation of up to ~0.05 Å. These non-linearities are due to non-linear imaging distortions of the HRC-S detector. We have derived and applied a preliminary wavelength correction as a function of detector location for data on the central HRC-S plate. The dispersion relation shows that the RMS decreases to 0.009 Å once the correction is applied. This is, however, work in progress, and test software is not yet available.

Observations

Our main calibration source for the dispersion relation is Capella, which is an active binary whose spectrum consists of many bright and narrow emission lines. This makes Capella an ideal target for calibrating the dispersion relation, since our analyses depend largely on being able to accurately measure the wavelength centroids of known spectral lines. The following table summarizes the primary observations used in our analyses:

Table 1: Summary of LETG+HRC-S Dispersion Relation Calibration Observations

Source	Obs ID	UT Start	Exposure [ks]	Offset [arcmin]
Capella	1248	1999 Nov 9 13:27:21	85.23	0
Capella	2582 >	2002 Oct 4 23:57:53	28.83	-1.5
Capella	3479	Oct 6 10:01:58	30.38	+1.5

Dispersion Relation

The plot below shows the dispersion relation, based on the measured wavelengths of emission lines from the Capella spectrum(ObsID 1248). The data here have been reprocessed with CIAO 3.1, which includes an adjustment for the plate gap sizes.

Figure 1: Measured minus theoretical wavelengths shown as a function of theoretical wavelength for Capella ObsID 1248. The green dashed lines represent the error weighted mean for each HRC-S microchannel plate.

Note that the error-weighted mean (green dashed lines) for the central and positive order plates are significantly offset from zero. This is not a systematic wavelength offset which needs to be adjusted for the 2 plates, but rather an effect of the non-linearities of the dispersion relation. Although non-linearities are prominent, the RMS of the observed minus reference wavelengths is only 0.014 Å across all wavelengths. The RMS across the central plate data is even smaller at 0.012 Å.

Mapping out non-linearities

A very simple and direct way to visualize the non-linearities of the dispersion relation across the detector is to plot photon events in λ versus tdety space, where tdety is the detector coordinate in the dispersion direction. For observations with normal mode pointing, the telescope dithers in a lissajous pattern, spreading out the photons along the detector, in both the dispersion and cross-dispersion directions. The width of the dither pattern is nominally ~300 pixels, or 40 arcseconds. We can use this dither pattern to probe the dispersion relation non-linearities across the detector.

Figure 2 shows the Fe XVII emission line from standard processed Capella data (red) plotted in λ versus tdety space. By measuring the wavelength centroids of the events as a function of tdety, we were able to obtain a wavelength correction which could be applied to individual photon events based on their tdety locations. Wavelength centroids were measured by splitting up the data into a number of tdety bins, then creating a wavelength histogram of the events in each bin, and fitting a modified Lorentzian function to the histograms. We then subtract the known laboratory or theoretical wavelength of the relevant line from the measured wavelength centroid of the data in each tdety bin, and obtain a wavelength correction as a function of detector location. Data shown in black in Figure 2 are photon events whose wavelengths have been shifted based on measured line centroids of the original data (red). The corrected data now appear to follow a much more horizontal line across the detector. (For details see SPIE paper by Chung et al., 2004.)

Figure 2: Data from the Fe XVII emission line shown in wavelength versus detector coordinate tdety (dispersion direction). In red, we show standard processed data (which has been vertically offset on the figure for clarity). Notice how these events ``wobble'' across the detector non-linearly. In black, we show the same data which have had wavelength corrections applied to them. The corrected data (black) show significant improvement, and follow a much straighter path across tdety as compared to the original data (red).

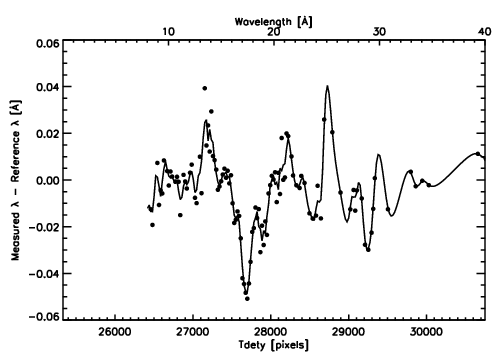
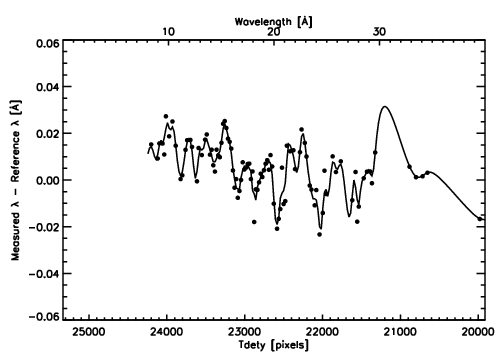
Although this method works very well in the example shown in Figure 2, there are some serious limitations to this method. Because the wavelength corrections are based on splitting up photons into bins along tdety, then measuring wavelength centroids, it is essential that we have relatively unblended, high signal-to-noise lines. Therefore, we can only obtain wavelength corrections for those regions on the detector where high S/N lines fall. In October of 2002, two separate observations of Capella were obtained, each with offset pointings of 1.5 arcminutes in opposite directions (ObsID 2582 and 3479). These offset pointing observations were planned such that bright lines would fall on different parts of the detector, so that we could probe a greater region of the detector for wavelength non-linearities. By using data from on-axis Capella observations, combined with these off-axis observations, we have derived a somewhat more complete wavelength correction as a function of tdety. This correction is shown in the Figure below.

Figure 3: Measured minus reference wavelength as a function of tdety, based on data from ObsIDs 1248, 2582, and 3479. The circles represent the measured wavelength minus reference wavelength for a given detector location, and the smooth black curve is a spline fit to the circles. The top and bottom plots show the wavelength correction for negative order and positive order spectra, in the wavelength range of approximately 7 to 40 Å.

Results

With the wavelength corrections shown in Figure 3 applied to Capella ObsID 1248 data, we see from Figure 4 that the non-linearities in the dispersion relation for data on the central plate flatten out significantly. The RMS across all wavelengths has now decreased to 0.009 Å, as compared to 0.014 Å for standard processed data (Figure 1), which do not yet include a correction for wavelength non-linearities.

Figure 4: The dispersion relation for Capella ObsID 1248, after corrections were applied to flatten out wavelength non-linearities. The RMS across all wavelengths is 0.009 Å.

The wavelength non-linearities that are currently observed in the data can broaden out line profiles quite significantly. Figure 5 is one of the most extreme examples of how much improvement we can expect to see in line profiles, once a correction is applied to flatten out the wavelength distortions. The post-correction data (red) has a much sharper peak, and narrower line width. In this case, the measured FWHM of the corrected data is approximately 25% smaller than the original data.

Figure 5: Fe XVII line profile from negative order Capella data. In black we show standard processed data, and in red we show data that have been wavelength corrected.

Although we have certainly made progress on characterizing the dispersion relation non-linearities along the detector, there still remain vast regions of the detector for which we do not have sufficient data to map out the wavelength distortions. We are expecting another observation of Capella, with an off-axis pointing of +3.0 arcminutes. This will allow us to characterize the wavelength non-linearities in regions of the detector that are currently unknown.

Future Work

The HRC-S degap and the observed wavelength distortions are related problems. Preliminary work has shown that by applying an empirical degap map to the data does improve the distortions in some areas of the detector, though not in others. In the future, we will attempt an iterative approach, in which we apply wavelength corrections based on the methods described above, then re-derive a new degap map, apply the new degap map, and re-derive the wavelength corrections, etc., until results from the wavelength distortions and degap converge.

Last modified: 11/18/11

Chandra Science | Chandra Home | Astronomy links | iCXC (CXC only) | Search

The Chandra X-Ray Center (CXC) is operated for NASA by the Smithsonian Astrophysical Observatory. 60 Garden Street, Cambridge, MA 02138 USA.    Email: cxcweb@head.cfa.harvard.edu Smithsonian Institution, Copyright © 1998-2004. All rights reserved.